 Originated
by the French mathematician Rene Thom in the 1960s, catastrophe theory is
a special branch of
dynamical systems theory
. It studies and classifies phenomena characterized by
sudden shifts in behavior arising from small changes in circumstances.
Originated
by the French mathematician Rene Thom in the 1960s, catastrophe theory is
a special branch of
dynamical systems theory
. It studies and classifies phenomena characterized by
sudden shifts in behavior arising from small changes in circumstances.
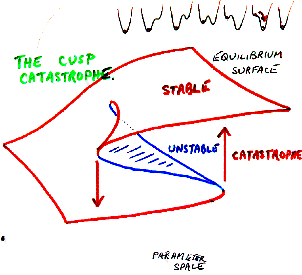
Catastrophes are
bifurcations
between different equilibria, or fixed point attractors.
Due to their restricted nature, catastrophes can be classified based on
how many control parameters are being simulataneously varied. For example,
if there are two controls, then one finds the most common type, called a
"cusp" catastrophe. If, however, there are move than five
controls, there is no classification.
Catastrophe theory has been applied to a number
of different phenomena, such as the
stability
of ships at sea and their capsizing
, bridge collapse,
and, with some less convincing success,
 the fight-or-flight behavior of animals and prison riots.
the fight-or-flight behavior of animals and prison riots.
|
